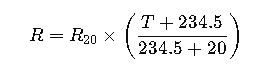Transformers serving non-linear loads face unique challenges due to harmonic currents causing additional heating. Calculating the K-Factor is essential for ensuring transformer reliability and longevity under such conditions.
This article explores the K-Factor calculation for non-linear loads based on IEEE C57 and IEC standards. It covers formulas, tables, and real-world examples to guide engineers in transformer selection and design.
Artificial Intelligence (AI) Calculator for “K-Factor for Non-Linear Loads in Transformers Calculator – IEEE C57, IEC”
- Calculate K-Factor for a transformer with 5th and 7th harmonic currents at 20% and 15% respectively.
- Determine K-Factor for a non-linear load with harmonic currents: I1=100A, I3=10A, I5=15A, I7=5A.
- Find K-Factor for a transformer feeding a variable frequency drive with harmonic distortion of 30% THD.
- Compute K-Factor for a transformer with harmonic currents I1=50A, I3=12A, I5=8A, I7=6A, I9=4A.
Comprehensive Tables of K-Factor Values for Non-Linear Loads
The K-Factor quantifies the additional heating effect caused by harmonic currents in transformers. It is crucial for selecting transformers that can withstand non-linear load stresses without premature failure. Below are detailed tables showing typical K-Factor values for various non-linear load types and harmonic current distributions, based on IEEE C57.110 and IEC 60076-7 standards.
| Load Type | Dominant Harmonics | Typical Harmonic Current % of Fundamental | Approximate K-Factor | Reference Standard |
|---|---|---|---|---|
| Computer Data Centers | 3rd, 5th, 7th | 3rd: 15%, 5th: 20%, 7th: 10% | 3.5 – 4.0 | IEEE C57.110 |
| Variable Frequency Drives (VFDs) | 5th, 7th, 11th | 5th: 25%, 7th: 15%, 11th: 5% | 4.0 – 5.5 | IEC 60076-7 |
| UPS Systems | 3rd, 5th, 7th | 3rd: 10%, 5th: 15%, 7th: 10% | 3.0 – 3.8 | IEEE C57.110 |
| Arc Furnaces | 3rd, 5th, 7th, 9th | 3rd: 30%, 5th: 25%, 7th: 20%, 9th: 10% | 5.0 – 6.5 | IEC 60076-7 |
| Fluorescent Lighting | 3rd, 5th | 3rd: 8%, 5th: 5% | 2.0 – 2.5 | IEEE C57.110 |
| Industrial Drives | 5th, 7th, 13th | 5th: 20%, 7th: 15%, 13th: 5% | 4.0 – 5.0 | IEC 60076-7 |
These values serve as guidelines for transformer manufacturers and electrical engineers to specify transformers with appropriate K-Factor ratings. Transformers with higher K-Factor ratings are designed with enhanced thermal capabilities to handle harmonic-induced heating.
Fundamental Formulas for Calculating K-Factor in Transformers
The K-Factor is a dimensionless multiplier that quantifies the additional heating effect caused by harmonic currents flowing through a transformer. It is defined as the ratio of the total harmonic losses to the losses caused by the fundamental frequency current alone.
The general formula for K-Factor is:
Where:
- K = K-Factor (dimensionless)
- Ih = RMS current of the hth harmonic
- I1 = RMS current of the fundamental frequency (h=1)
- h = harmonic order (integer, e.g., 1, 3, 5, 7, …)
This formula sums the squared ratio of each harmonic current to the fundamental current, weighted by the square of the harmonic order. The higher the harmonic order and magnitude, the greater the contribution to the K-Factor.
Expanded Explanation of Variables
- Ih: The RMS current magnitude of the harmonic component at order h. For example, I3 is the RMS current of the 3rd harmonic.
- I1: The RMS current magnitude of the fundamental frequency (usually 50 Hz or 60 Hz).
- h: The harmonic order, an integer multiple of the fundamental frequency.
Note that the fundamental current (h=1) contributes a value of 1 to the summation since (I1/I1)2 × 12 = 1.
Alternative Formulation Using Harmonic Current Magnitudes
Sometimes, the K-Factor is expressed as:
This highlights that the fundamental frequency contributes a baseline of 1, and the harmonics add additional heating effects.
Interpretation of K-Factor Values
- K = 1: Purely linear load, no harmonics present.
- K > 1: Presence of harmonics causing additional heating.
- K ≈ 3 to 6: Typical range for transformers feeding non-linear loads.
Step-by-Step Real-World Examples of K-Factor Calculation
Example 1: Calculating K-Factor for a Transformer Feeding a Computer Data Center
A transformer supplies a computer data center with the following harmonic current components (RMS values):
- I1 = 100 A (fundamental)
- I3 = 15 A (3rd harmonic)
- I5 = 20 A (5th harmonic)
- I7 = 10 A (7th harmonic)
Calculate the K-Factor for this transformer.
Solution:
Step 1: Calculate the ratio of each harmonic current to the fundamental current:
- (I3 / I1) = 15 / 100 = 0.15
- (I5 / I1) = 20 / 100 = 0.20
- (I7 / I1) = 10 / 100 = 0.10
Step 2: Square each ratio and multiply by the square of the harmonic order:
- 3rd harmonic: (0.15)2 × 32 = 0.0225 × 9 = 0.2025
- 5th harmonic: (0.20)2 × 52 = 0.04 × 25 = 1.0
- 7th harmonic: (0.10)2 × 72 = 0.01 × 49 = 0.49
Step 3: Sum all harmonic contributions and add 1 for the fundamental:
Therefore, the K-Factor is approximately 2.69, indicating the transformer must be rated to handle nearly 2.7 times the heating caused by the fundamental current alone.
Example 2: K-Factor Calculation for a Transformer Feeding a Variable Frequency Drive (VFD)
A transformer feeds a VFD with the following harmonic currents:
- I1 = 80 A
- I5 = 20 A
- I7 = 12 A
- I11 = 5 A
Calculate the K-Factor.
Solution:
Step 1: Calculate harmonic ratios:
- 5th: 20 / 80 = 0.25
- 7th: 12 / 80 = 0.15
- 11th: 5 / 80 = 0.0625
Step 2: Calculate weighted squares:
- 5th: (0.25)2 × 52 = 0.0625 × 25 = 1.5625
- 7th: (0.15)2 × 72 = 0.0225 × 49 = 1.1025
- 11th: (0.0625)2 × 112 = 0.00390625 × 121 = 0.4727
Step 3: Sum and add 1:
The K-Factor is approximately 4.14, indicating significant harmonic heating effects requiring a transformer designed for K4 or higher.
Additional Technical Considerations for K-Factor Transformers
Transformers designed for non-linear loads with elevated K-Factors incorporate several design enhancements to mitigate harmonic heating:
- Increased conductor cross-section: To reduce I²R losses from harmonic currents.
- Improved cooling systems: Enhanced oil flow or forced air cooling to dissipate heat.
- Special core materials: To reduce eddy current losses at harmonic frequencies.
- Reduced winding impedance: To minimize voltage distortion and losses.
IEEE C57.110 and IEC 60076-7 provide guidelines for specifying transformers with appropriate K-Factor ratings, ensuring safe operation under harmonic-rich environments.
Summary of IEEE C57 and IEC Standards on K-Factor Transformers
| Standard | Scope | K-Factor Range | Key Provisions |
|---|---|---|---|
| IEEE C57.110 | Guide for Transformers Subjected to Non-Sinusoidal Load Currents | 1 to 30 | Defines K-Factor calculation, transformer design recommendations, and testing procedures. |
| IEC 60076-7 | Power Transformers – Part 7: Loading Guide for Transformers | 1 to 30 | Provides loading guidelines considering harmonic currents and thermal effects. |
Both standards emphasize the importance of accurate harmonic current measurement and K-Factor calculation to prevent transformer overheating and premature failure.
Practical Tips for Engineers Using K-Factor Calculations
- Measure harmonic currents accurately: Use true RMS meters or harmonic analyzers to capture harmonic spectrum.
- Consider all significant harmonics: Typically up to the 13th or 15th harmonic for industrial loads.
- Use conservative K-Factor values: Account for load growth and measurement uncertainties.
- Specify transformers with appropriate K-Factor ratings: Consult manufacturers for transformers rated K4, K13, or higher as needed.
- Monitor transformer temperature: Use thermal sensors to detect overheating due to harmonics.
Adhering to these practices ensures transformer reliability and reduces downtime in facilities with non-linear loads.
References and Further Reading
- IEEE C57.110-2014 – Guide for Transformers Subjected to Non-Sinusoidal Load Currents
- IEC 60076-7: Power Transformers – Part 7: Loading Guide for Transformers
- NEMA Standards for Transformers
- Eaton – K-Factor Transformers Overview